Core Math Worksheets
Fraction Worksheets
Word Problems
Algebra and Trigonometry
Other Worksheets
Place Value
Percentages
Rounding Numbers
Ordering Numbers
Standard, Expanded, Word Form
Mean Median Mode Range
Ratio Worksheets
Probability Worksheets
Roman Numerals
Factorization, GCD, LCM
Prime and Composite Numbers
Pre-Algebra
Geometry Worksheets
Blank Clocks
Telling Analog Time
Analog Elapsed Time
Greater Than and Less Than
Money
Arithmetic Sequences
Geometric Sequences
Venn Diagram
Graph Worksheets
Measurement & Conversions
Patterns and Puzzles
Color by Number
Holiday & Seasonal
Early Learning
Printables
Calculators
Factoring Polynomials Worksheets
Factoring polynomials using greatest common factor, grouping, binomial factoring and more! Includes specific practice for both quadratic and linear expressions.
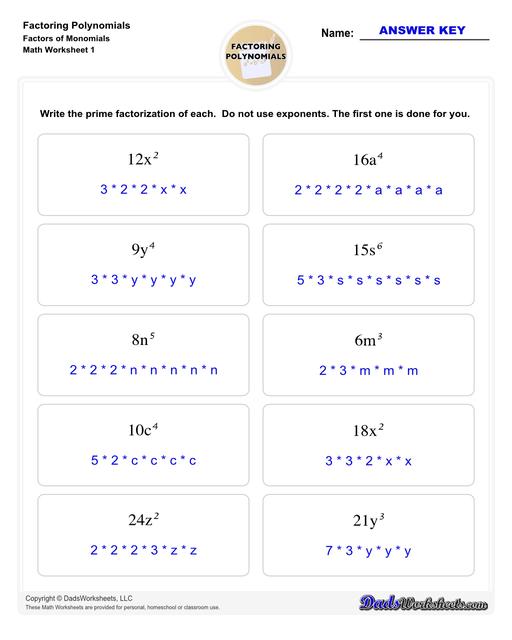
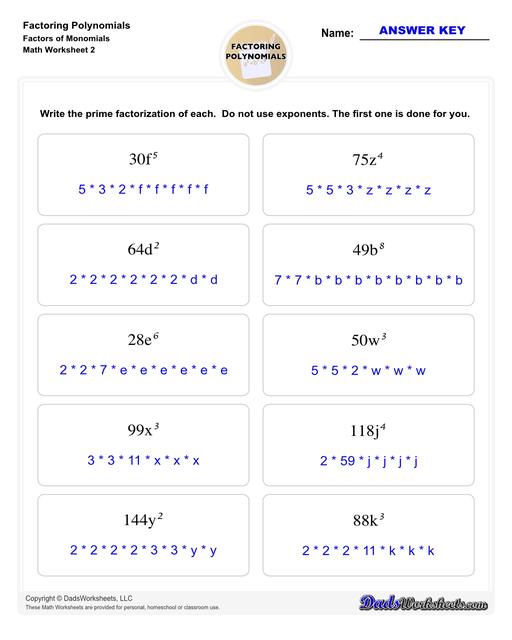
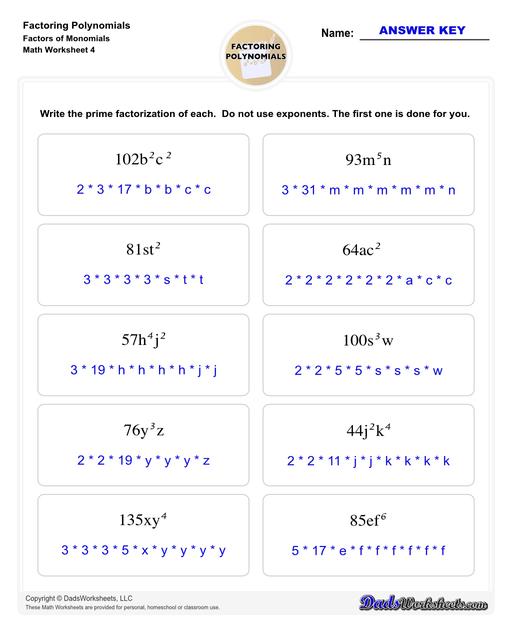
Factors of Monomials Worksheets
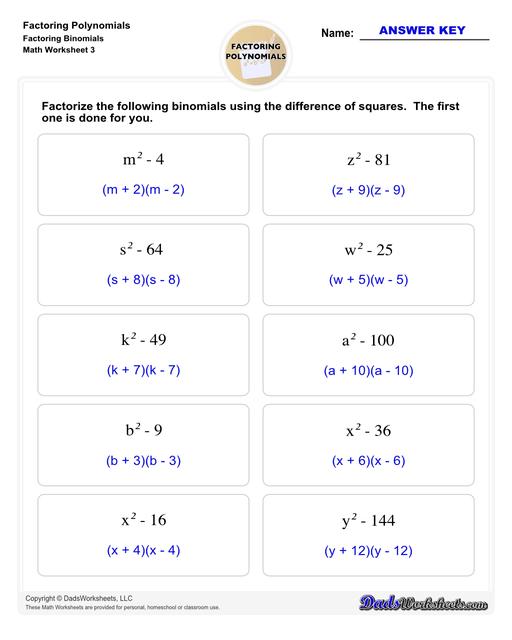
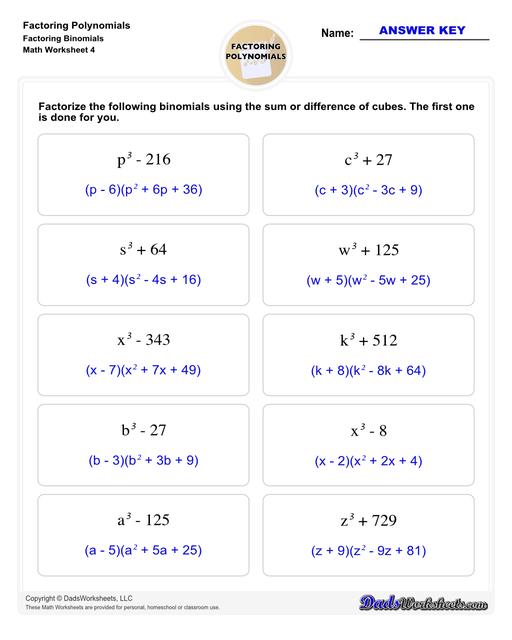
Factoring Binomials Worksheets
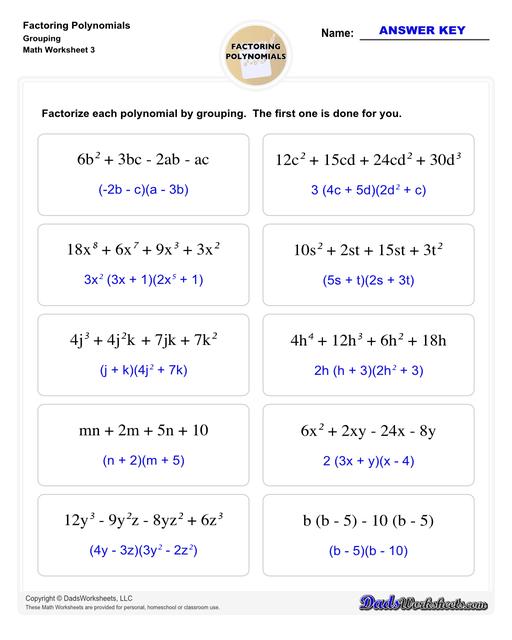
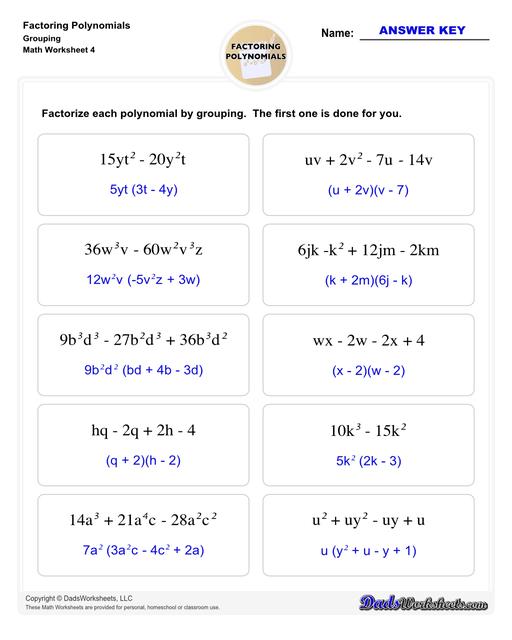
Grouping Polynomial Terms Worksheets
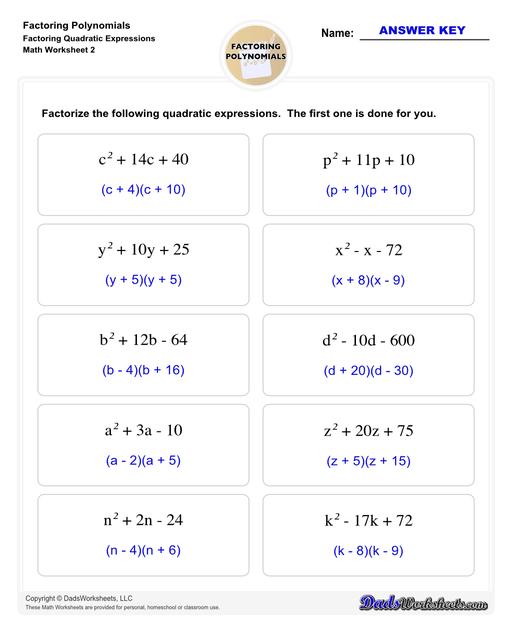
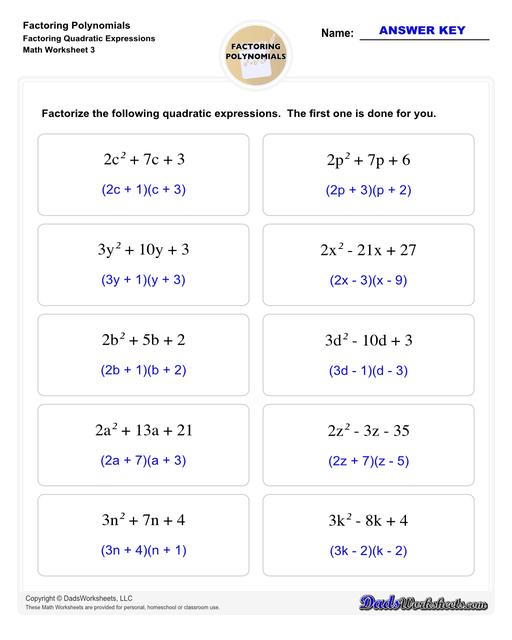
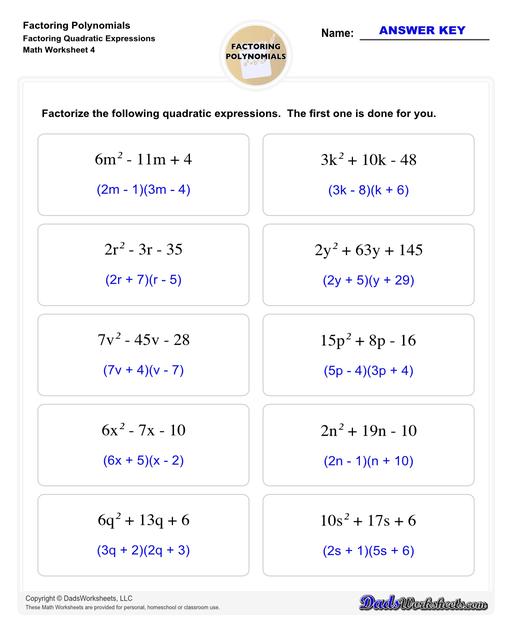
Factoring Quadratic Expressions Worksheets
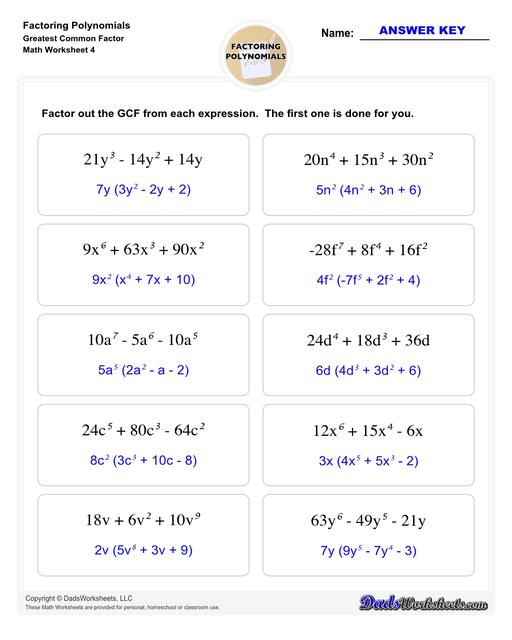
Greatest Common Factor Polynomial Worksheets
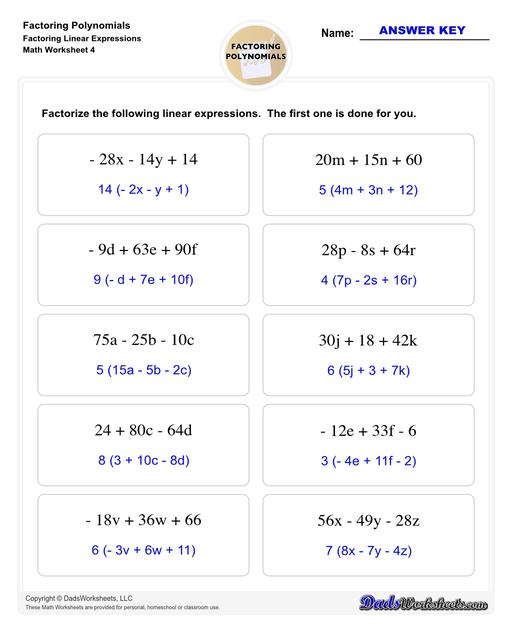
Factoring Linear Expressions Worksheets
Factoring Polynomials
The factoring polynomials worksheets on this page will help students understand the concept and identify different techniques for factoring polynomials. There are six sets of worksheets available that covers six methods for factoring polynomials: Factors of Monomials; Factoring using the Greatest Common Factor (GCF); Factoring by Grouping; Factoring Binomials; Factoring Quadratic Expressions; and Factoring Linear Expressions. All of these worksheets include answer keys! Go ahead and check out these factoring polynomials worksheets or continue reading below and let’s delve together into the world of polynomial factoring.
Parts of a Polynomial
The polynomial definition is in fact straightforward. The prefix "Poly" means "many" and “Nominal” means terms. But how do you tell when you're working with polynomials? And how do you explain the different components in a polynomial?
First, let’s learn the different parts of polynomials. Refer to the images below…

Terms: These are the basic components of a polynomial. Each term consists of a coefficient multiplied by one or more variables raised to non-negative integer exponents and are generally separated by “+” or “-” signs. In our example above, the terms are 3x^2, 2y, and -1.
Operator: Any symbol that indicates an operation to be performed. For example: + for addition; − for subtraction.
Coefficients: These are the numerical factors that are multiplied by the variables in each term. To get polynomial coefficients, take the numbers in front of each terms. The coefficients in our example are 3, 2, and -1.
Variables: These are the symbols that represent unknown values and can be raised to non-negative integer exponents. The variables in our example are x and y.
Constant: This is the term without any variables, often represented by just a numerical value. In our given example, the constant term is -1.
Exponents: These are the powers to which variables are raised. Keep in mind that if the variable has no exponent, it actually has an exponent of 1. It the term does not have variable, it is degree 0. In the polynomial shown above, 2 is the exponent of x in the first term, and 1 is the exponent of y in the second term.
Degree: The degree of a polynomial is the highest exponent of its variable(s) when the polynomial is written in standard form. Refer to the image above. The exponent of variable x is 2 and 1 in variable y, thus the degree of the polynomial is 2.
Types of Polynomials
Generally, polynomials are written with its terms being ordered in decreasing order of exponents. In other words, the terms are arranged in descending order of the exponents of a single variable, starting from the highest exponent and moving down to the lowest.
Although polynomials can range from one to a very large amount of terms, you may hear specific names referencing to polynomials of a set number of terms. These polynomials are classified based on the number of terms they contain. Here are the main types based on the number of terms:
Monomial: one-term polynomial (e.g. 3x)
Binomial: two-term polynomial (e.g. 3x + 2y)
Trinomial: three-term polynomial (e.g. 3x + 2y - 1)
Quadrinomial: polynomial with four terms (e.g. 4x + 2y + x + 2)
Multinomial: polynomial with more than four terms (e.g. 4x + 2y + 2x + y + 2)
How To Write Polynomials
Writing polynomials involves expressing algebraic expressions that consist of variables and constants combined using arithmetic operations. Here's a step-by-step guide on how to write polynomials:
Step 1: Identify the Terms: Look at the given problem or expression and identify each term. Terms consist of a coefficient (a numerical factor) and one or more variables raised to non-negative integer exponents.
Step 2: Arrange the Terms: Write down the terms in the desired order. Usually, the term with the largest exponent goes first, followed by the term with the next highest exponent and so on till you reach a constant term.
Step 3: Combine Like Terms: Terms that have the same variable(s) raised to the same exponent(s) can be combined. Combine coefficients by adding or subtracting them.
Step 4: Write the Expression: After arranging and combining like terms, write down the expression. Ensure that each term is separated by addition or subtraction signs.
Step 5: Specify the Degree: Determine the degree of the polynomial, which is the highest exponent of the variable(s) in the expression.
Here's an example of writing a polynomial:

This polynomial consists of four terms and rewritten in descending order. It involves the variable x raised to the power of 2. There are like terms to combine (4x and -2x). The degree of the polynomial is 2.
Remember, the key to writing polynomials is to understand the terms, arrange them properly, combine like terms if possible, and specify the degree of the polynomial.
How To Factor Polynomials
Polynomial factoring involves expressing a polynomial as a product of simpler polynomials or monomials. The fundamental theorem of algebra states that any polynomial of degree 'n' can be factored into 'n' linear or irreducible polynomial factors. Let's discuss the different techniques to factor polynomials.
Factors of Monomials: As mentioned above, a monomial is a one-term polynomial and factoring monomials is a technique used to factor a monomial into smaller terms or express it as a product of two or more terms. To factor monomials, you need to rewrite it with its separate parts all written out. This means expanding the variables with exponents and performing prime factorization on the coefficients. Here’s how:

Factoring Using The Greatest Common Factor: This technique involves factoring out the greatest common factor (GCF) from all the terms of the polynomial. The GCF for a polynomial is the largest monomial that is a factor of (divides) each term of the polynomial. To do this, first look at the coefficients and find the greatest number that can be divided into all evenly. Next, check if there is a variable present in every term. Lastly, identify the GCF by combining the common coefficient and variable in every term. Take a look at the illustration below:

The greatest number that you can divide evenly in every term is 2, and there is a common variable present among all terms, which is two x’s. Hence, the GCF for this polynomial is 2x^2 as shown in the answer.
Factoring by Grouping: This technique is used in cases when there is no common factor in all the terms of a polynomial. In this method, we group the terms of the polynomial and look for common factors within each group. Then look for the GCF in each part. This method is typically used when a polynomial has four or more terms. Here's an example to illustrate the process:

After factoring out any common factors, you should have a simplified expression. If possible, continue factoring until you can no longer factor. You can check your solution by multiplying the factors together to obtain the original polynomial expression.
That's the basic idea behind factoring polynomials by grouping. It's a useful technique for simplifying polynomial expressions, especially when they have four or more terms.
Factoring Quadratic Expressions: Quadratic expression is an algebraic statement with a power of two being its highest term and these type of polynomial can be factored using methods like decomposition or completing the square. Here’s an example…

Factoring Linear Expressions: Linear expression is an algebraic statement where none of the exponents are greater than 1 and these type of polynomial can be factored by finding its greatest common factor or the GCF. Here’s an example…