Multiplying Signed Numbers

These worksheets practice multipling two or three signed numbers, as well as zero. The problems on these worksheets help students understand how to determine whether the product of a multiplication is positive, negative or zero, and also provide practice determining multiplicands (and their signs) from the product.

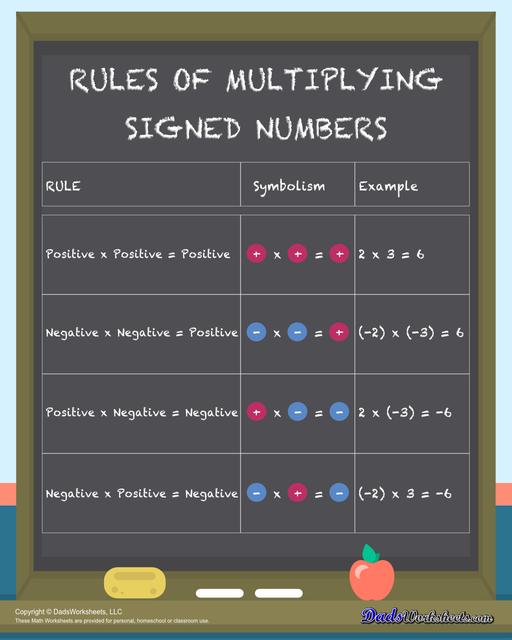
Rules for Multiplying Signed Numbers

Learning the basics of multiplying integers is an essential step in understanding arithmetic operations involving positive and negative numbers. Consistent practice is key to mastering the basics of multiplying integers and the worksheets on this page will help you do just that! There are two sets of worksheets for multiplying signed numbers available ~ multiplying two integers and multiplying three integers. Both sets have variation of problems that are perfect for practicing your multiplication skills on signed numbers. Use this Rules of Multipying Signed Numbers Chart as a guide when you are just starting to learn the rules.

Multiplying Two Signed Integers

Integers are whole numbers that can be positive, negative, or zero. Positive integers are greater than zero, while negative integers are less than zero. Zero is considered neither positive nor negative. Multiplying signed integers involves applying specific rules to determine the sign of the product based on the signs of the factors involved. Whether the numbers are positive or negative dictates the outcome of the multiplication. Understanding these rules is essential for performing arithmetic operations accurately, especially in algebra and other mathematical contexts. Let us learn the basics together…

Remember the following rules:

• The product of two positive numbers is positive.
• The product of two negative numbers is positive.
• The product of a positive and a negative number (or vice versa) is negative.

The chart below will help you remember the rules for multiplying integers:

Take a look at the examples below…

Positive × Positive:

When multiplying two positive numbers, the product is always positive. This rule reflects the idea that combining two quantities of the same sign results in a larger quantity.

Both numbers are positive so the answer is positive.

Negative × Negative:

Similarly, when multiplying two negative numbers, the product is also positive. This rule follows from the concept that multiplying two opposite quantities results in a larger quantity.

Even if the numbers are both negative, since the two signs are the same, the answer is still positive.

Positive × Negative or Negative × Positive:

Multiplying a positive number by a negative number or vice versa yields a negative product. This rule stems from the concept of combining opposite quantities, which results in a smaller quantity.

In this case, the signs are not the same so the answer is negative. The order doesn’t matter here.

Zero:

Multiplying any number by zero results in zero, regardless of the sign of the number. This rule is consistent with the concept that any quantity multiplied by zero equals zero.

Multiplying Three or More Signed Integers

Multiplying three or more integers follows the same principles as multiplying two integers. You simply multiply all the integers together, keeping track of the signs, and then determine the sign of the product based on the number of negative factors involved.

Here's how you can approach multiplying three or more integers:

Step 1: Multiply all the integers together: Simply multiply all the integers together, disregarding their signs at this point.

Step 2: Count the number of negative factors: Count how many of the integers being multiplied are negative.

Step 3: Determine the sign of the product according to the multiplication of integers rules.

• If the number of negative factors is even (including zero), the product is positive.
• If the number of negative factors is odd, the product is negative.

Let's illustrate this with examples:

Example 1: Multiply the integers (−2) × (−3) × (+4)

Multiply all the integers together (disregarding their signs):

(−2) × (−3) × (+4) = 24

Count the number of negative factors:

In this case, there are two negative factors: (−2) and (−3)

Determine the sign of the product:

Since there are two negative factors (an even number), the product is positive.

Therefore, (−2) × (−3) × (+4) = (+6) × (+4) = +24.

Example 2: Multiply the integers (−2) × (−3 ) × (+4) × (−5)

Multiply all the integers together. Again, disregarding their signs at this point:

(−2) × (−3) × (+4) × (−5) = 120

Count the number of negative factors:

In this case, there are three negative factors: (−2), (−3), (−5).

Determine the sign of the product:

Since there are three negative factors (an odd number), the product is negative.

Therefore, (−2) × (−3) × (+4) × (−5) = −120.

In summary, when multiplying three or more integers, multiply them together and then determine the sign of the product based on the number of negative factors involved, following the rule that an odd number of negative factors results in a negative product, and an even number (including zero) results in a positive product.

Just like any other rules in mathermatics, these rules of multiplying signed numbers has practical applications in various fields. By mastering these rules, individuals can accurately perform arithmetic operations involving positive and negative numbers, enabling them to solve complex problems efficiently and interpret real-world scenarios effectively.